Welcome to the realm of linear equations, where the concept of slope plays a pivotal role in understanding the behavior of lines. This comprehensive guide, unit 4 linear equations homework 1 slope answer key, serves as your trusty companion on this mathematical journey, providing insightful explanations, practical examples, and expert solutions to equip you with a solid grasp of slope and its applications.
Throughout this exploration, we will delve into the intricacies of calculating slope, uncovering the different types of slopes and their graphical representations. We will master the art of graphing linear equations using slope and y-intercept, unlocking the power of slope in real-world applications, and unravel the mysteries of parallel and perpendicular lines.
Linear Equations Homework 1: Slope

This homework assignment covers the concept of slope in linear equations. Students will learn how to calculate slope, identify different types of slopes, graph linear equations using slope, and apply slope to real-world situations.
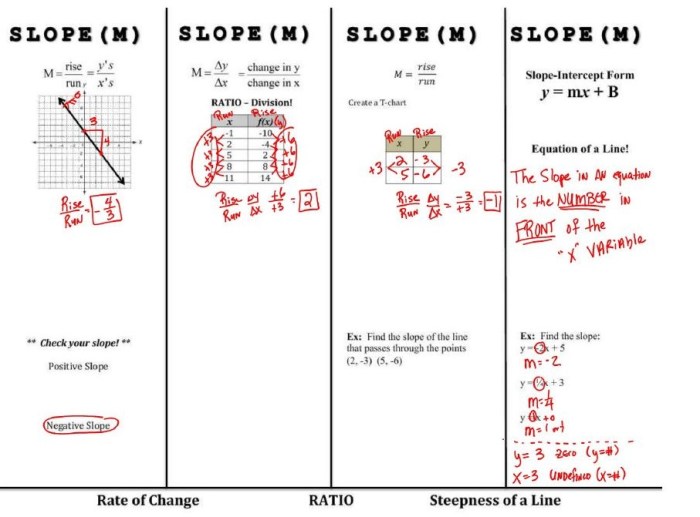
Slope Formula
The slope of a line is a measure of its steepness. It is calculated using the following formula:
$$m = \fracy_2
- y_1x_2
- x_1$$
where \(m\) is the slope, \((x_1, y_1)\) and \((x_2, y_2)\) are two points on the line.
Examples:
- For the line passing through the points \((1, 2)\) and \((3, 6)\): $$m = \frac6 – 23 – 1 = \frac42 = 2$$
- For the line passing through the points \((-2, 5)\) and \((0, 1)\): $$m = \frac1 – 50 – (-2) = \frac-42 = -2$$
Types of Slopes, Unit 4 linear equations homework 1 slope answer key
There are four main types of slopes:
- Positive slope:A line with a positive slope rises from left to right. The slope is greater than 0.
- Negative slope:A line with a negative slope falls from left to right. The slope is less than 0.
- Zero slope:A line with a zero slope is horizontal. The slope is 0.
- Undefined slope:A line with an undefined slope is vertical. The slope is undefined.
Examples:
- The equation \(y = 2x + 1\) has a positive slope of 2.
- The equation \(y = -3x + 4\) has a negative slope of -3.
- The equation \(y = 5\) has a zero slope.
- The equation \(x = 2\) has an undefined slope.
Key Questions Answered: Unit 4 Linear Equations Homework 1 Slope Answer Key
What is the slope formula?
The slope formula is m = (y2 – y1) / (x2 – x1), where (x1, y1) and (x2, y2) are two points on the line.
What are the different types of slopes?
There are four types of slopes: positive, negative, zero, and undefined.
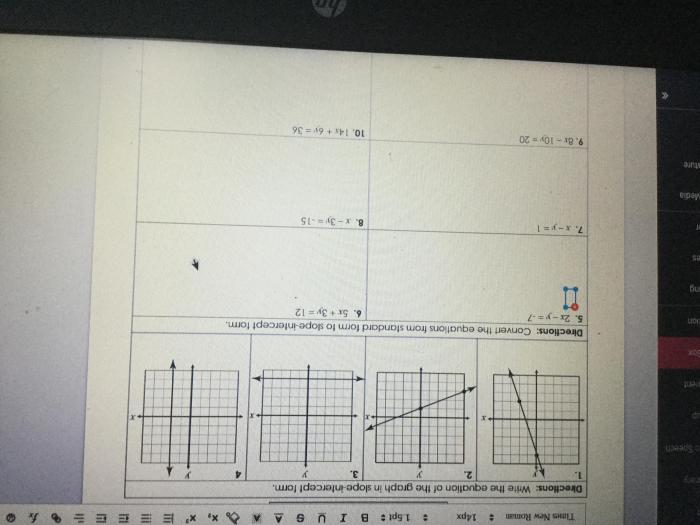
How do you graph a linear equation using slope and y-intercept?
To graph a linear equation using slope and y-intercept, first plot the y-intercept on the y-axis. Then, use the slope to find a second point on the line. Draw a line through the two points.